May_07_article03

Editor’s Note: This research uses a pretest, post-test and delayed test to determine the efficacy of Computer Assisted Learning.

Computer Assisted Learning (CAL) Strategy
for “Numerical Methods Course” - an Experiment

Satish Rastogi, Ashok S. Pawar

India

Facilitating the learner to learn is very much associated with the efforts of a teacher to adapt the process for a specific learner. Creating an interest and active involvement with the learner is the responsibility of the teacher. In the words of Jack Koumi “If the teacher can create an enduring fascination for the subject matter, the job is almost over: the more the students love the subject, the less help they need in their studies.” The strategy to be used should be affective or motivational rather than cognitive.

Computers are used in almost every aspect of daily life, and its widespread use has proved the tremendous potential of computer technology for helping mankind. Many experiments have been conducted in India to assess the effectiveness of computer technology in learning. Richard A. Shade and M. N. G. Mani, N. S. Yemul, K. K. Soni, Narayan Ugar, D. Ray, M. Chandwani Abhay Jain and S. Mukherjee, performed experiments to determine the effectiveness of computers in the teaching-learning process. Mukhopadhyay (1993) made a reference to Paliniapan V. P., Goyal D. R., Nachimuthu K., Subramaniam K., Malathi Rao & Usha Rao, Anil Kumar, Rastogi Rashmi, Mitra S., Gupta Madhu, Pant M.M., for developing computer strategies to learn different academic course content. These experiments enlightened investigators to develop the Computer Assisted Learning (CAL) strategy for degree students to learn the concept of Numerical Methods.

Learning Difficulties in Numerical Methods

The widespread use of digital computers in recent years in scientific research has made the study of Numerical Methods as important as the study of Mathematics. A number of Universities have introduced the course ‘Numerical Methods’ in the undergraduate science curricula. Learning tedious and difficult contents of Numerical Methods in the classroom is time consuming and boring. This creates apathy in the minds of learners and they try to ignore this content. Students require a process or technology to save their time and sustain a continuous interest among them. The investigators thought to take the advantage of CAL strategy and develop some software for learning Numerical Methods in an easier way.

Objectives of the Study

Hypotheses

Significance of the Study

The content of Numerical Methods is very important to undergraduate and postgraduate students for solving application problems in Physics, Electronics, and Computer Science as is evident from a course content analysis. The long calculations are not reliable, boring, and students develop apathy towards this content. An urgent need was to have an innovative approach to developing an effective CAL strategy for learning this content. This experimental research is also very significant because it opens the doors of computer applications for learning tedious and difficult course content. The National Council of Teachers of Mathematics in U.S.A. has very rightly justified the use of computer in the learning and teaching of Mathematics as

“Teachers should use computers as tools to assist students with the exploration and discovery of concepts, with the transition from concrete experiences to abstract mathematical ideas, with the practice of skills, and with the process of problem solving. In mathematics education, the computer must be instructional aids and not the object of instruction”.

Many researchers found that irrespective of the subject taught, the reasons for adopting CAL remain the same. The computer has been found to be useful as a means of (1) augmenting teaching/training methods, (2) accelerating the learning process, (3) experimenting in course development, (4) providing remedial instruction, (5) providing individualized instruction, (6) providing enrichment material, (7) achieving consistently higher teaching standards, (8) providing cost effective instruction, (9) providing ‘on demand’ instruction. This has motivated the investigators to see the effectiveness of CAL in learning Numerical Methods.

Limitations of the Study

This research is limited to students of undergraduate degree courses. Both males and females were selected for this study. Students from four disciplines: Physics, Electronics, Mathematics, and Computers were chosen for the purpose of comparison. This study was limited to the students of Sri Shivaji Vidya Prasarak Sanstha’s Science College, Dhule, Maharashtra (India) for the sake of convenience and constraints of time and money. This was a self financing project and so investigators decided to select their sample from Dhule town only. Numerical Methods is a very vast subject. Every method cannot be included for this research. The investigators have selected only the Iterative methods covering Bisection Method, False Position Method, Newton-Raphson Method for this project.

Research Methodology

(1) Population and Sample for the Study

This was an experimental research for assessing the effectiveness of CAL strategy to learn Numerical Methods. It was decided that the population for this study would be comprised of students studying Numerical Methods in Physics, Electronics, Computers and Mathematics at the undergraduate level in colleges affiliated with North-Maharashtra University, Jalgaon. At this time, there are twelve colleges where the ‘Numerical Methods’ course is taught at undergraduate level. Students studying this course at Shri Shivaji Vidya Prasarak Sanstha’s Science College, Dhule, Maharashtra, India were taken as sample for this study.

(2) Tools Development

(a) Development of Achievement Test: The content covering Bisection Method, False Position Method, and Newton-Raphson Method was analyzed and a questionnaire having five open type Questions for a total of one hundred marks was constructed. For this purpose, the investigators selected about fifteen questions and submitted them to five experts in the field of Numerical Methods. After a long discussion, ten questions were dropped and five questions were kept in the Questionnaire. Ten questions were dropped because, in the opinion of all five experts, they were too simple. The students were allotted two hours to answer this Questionnaire. This test was used as Pre-test, Post-test and Retention-test. Students were asked to use a computer to solve these questions under the supervision of the investigators.

(b) Development of CAL Software: The investigators analyzed the content and explained it using simple language, picture and examples. The script developed was discussed with five software experts. After implementing their valuable suggestions, the script was made final for loading in the computer. The software was developed in Visual Basic. Executable programs of Numerical Methods are written in C++ language. This loaded script (software) was reviewed by software experts and some corrections were made based on their advice. This software was used for the purpose of this project.

(c) Essay Writing – A Tool for Learners’ Opinion: The investigators requested students to write an essay covering their experiences and their opinion about the CAL strategy. These essays were examined to determine their opinion and experiences. Analysis revealed many common opinions; a few were specific. These were sorted to assess the acceptance of the CAL strategy.

The common points revealed that CAL was recognized as an approach to individualized instruction. Learners realized the importance of Computer Education. CAL can be used successfully for learning difficult Numerical Methods concepts. Educational Institutions will function differently if they use computer support for teaching and learning activities. CAL was considered to be cost effective. It helped learners to solve problems that were very difficult to solve manually. Once developed, CAL can be used for years by successive groups of students.

Specific problems were stated by some students. They complained that the cost of purchasing computers at their residence was beyond their means. They also noted frequent failure of the electricity supply in rural areas and their elementary (limited) knowledge of computer operations.

(3) Procedure

The investigators conducted a Pre-test and analyzed the student responses. Students were asked to study the CAL content and were assisted by the investigator with the help of computer for about one month. No time limit was imposed for learning so that students were able to learn at their own rate. After one month, a Post-test was carried out. It was followed by the Retention-test after two months. Students were asked to write an essay about their experiences and opinions regarding various activities of this experiment. These opinions were analyzed and conclusions were drawn. This methodology was followed on a single group design.

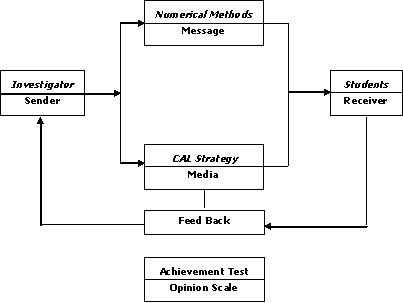
Figure 1. Experimental Design

Data Collection And Analysis

The marks obtained by the target group for Pre-test, Post-test and Retention-test were analyzed as per the objective of the study. There were forty four students taken in the sample. The distribution is shown below.

Table 1

Categorywise Distribution of Target Group (Total 44)

Group	Number of Students	Remarks
Electronics	10	Third Year - Bachelor of Science.
Computer	18	Third Year - Bachelor of Science.
Physics	11	Third Year - Bachelor of Science.
Mathematics	05	Third Year - Bachelor of Science.
Total	44

The marks obtained by the students were analyzed statistically The Mean, Standard deviation and t-values are given in Table-2.

Table 2

Statistical Analysis of Target Group Performance
(Total 44)

Table 2 reveals that there is significant difference in level of achievement between Pre-test and Post-test stages as well as between Pre-test and Retention-test stages because the ‘t’ value obtained is much higher than the standard table value 2.704. The difference is not significant between Post-test and Retention-test even at 0.05 level as the ‘t’ value obtained is much lower than the standard table value 2.021 .

The data indicated that the CAL strategy is very effective for learning Numerical Methods and resulted in significant change in the level of Knowledge. The Retention test showed that the target group retained almost similar level of information to the Post-test and so the forgetting factor was negligible (not significant).

Table 3

Statistical Analysis of Target Group Performance
[Electronics Group (Total : 10)]

The target group had ten students from Electronics. Analysis of the data obtained from Pre-test, Post-test, and Retention-test revealed that the difference between Pre-test and Post-test as well as between Pre-test and Retention-test was highly significant at 0.01 level. The ‘t’ value obtained is much higher than the standard table value 3.355. The difference is not significant between Post-test and Retention-test even at 0.05 level as the ‘t’ value obtained is much lower than the standard table value 2.306 . This analysis indicates that CAL strategy was effective with the target group (Electronics).

Table 4 represents the computer group with eighteen students. The data revealed, after analysis, that the difference in scores between Pre-test and Post-test as well as between Pre-test and Retention-test were highly significant at 0.01 level. It is because the ‘t’ value obtained is much higher than the standard table value 2.921 . The difference is not significant between Post-test and Retention-test even at 0.05 level as the ‘t’ value obtained is much lower than the standard table value 2.020. This analysis indicates that CAL strategy was found much effective with target group (Computer).

Table 4

Statistical Analysis of Target Group Performance.

[Computer Group (Total :18)]

Table5

Statistical Analysis Of Target Group Performance
[Physics Group (Total : 11)]

Data obtained from Pre-test, Post-test and Retention-test revealed, after analysis, that the difference of marks between Pre-test and Post-test as well as between Pre-test and Retention-test was highly significant at 0.01 level. This is because the ‘t’ value obtained is much higher than the standard table value 3.250. The difference is not significant between Post-test and Retention-test even at 0.05 level as the ‘t’ value obtained is much lower than the standard table value 2.282 . This analysis indicates that CAL strategy was found much effective with target group (Physics) also.

Table 6

Statistical Analysis Of Target Group Performance
[Maths Group (Total : 5)]

Data obtained from Pre-test, Post-test and Retention-test revealed, after analysis, that the difference of marks between Pre-test and Post-test as well as between Pre-test and Retention-test was highly significant at 0.01 level. It is because the ‘t’ value obtained is much higher than the standard table value 5.841. The difference is not significant between Post-test and Retention-test even at 0.05 level as the ‘t’ value obtained is much lower than the standard table value 3.182 . This shows that the CAL strategy was effective with target group (Math).

These tables indicate that CAL strategy was effective in assisting students in all target groups to learn Numerical Methods.

Table 7

Statistical Analysis of Performance of Learners in Subgroups

Table 8

Comparison of Performance of Learners in Subgroups

Tables 7 and 8 compare the performance of learners in various subgroups at three stages. Data analysis for Pre-test indicates that the difference between Electronics and Computer, Electronics and Physics, Electronics and Mathematics, Computer and Physics, Computer and Mathematics, Physics and Mathematics were not significant at 0.05 level. Hence performance of all four groups was identical in Pre-test. The data analysis for Post-test indicates that the difference between Electronics-Computer, Computer-Physics, Computer-Mathematics and Physics-Mathematics, groups were not significant even at 0.05 level. But, the difference between Electronics-Physics and Electronics- Mathematics were significant at 0.01 level.

Figure 2. Graphical presentation of learner performance in all four groups

Data analysis for the Retention-test indicates that the performance of all four groups was identical, because the difference found was not significant at 0.05 level. The essays, written by students about their experiences and opinions towards CAL witnessed that they enjoyed the CAL strategy and maintained high creativity, interest and motivation levels during the experiment. Some of them wanted similar strategies for the remaining content in Numerical Methods. The analysis indicates that the difference between Pre-test and Post-test was highly significant and so Hypothesis number one was not accepted. The difference in the level of achievement of the target group between Pre-test and Retention-test was highly significant, so Hypothesis number two was not accepted. The difference in the level of achievement of the target group between Post-test and Retention-test was not found significant even at 0.05 level and so Hypothesis number three was accepted. The opinion of the learners regarding CAL obtained through essays indicate that there was no controversy among them about their opinion and every body enjoyed and was interested in learning with the help of CAL strategy. Thus the Hypothesis number four was not accepted.

Conclusion

The CAL strategy developed by the investigator raised the level of achievement in Numerical Methods among third year Bachelor of Science (B.Sc.) students. It is recommended that similar strategies for other content be developed and the effectiveness be studied. This experiment opened the door for researchers to conduct similar experiments for other target groups in Numerical Methods content. It may also motivate the researchers to develop CAL software for other subjects.

Bibliography

Best J.W.(1977) : Research in Education, 3^rd Edition, Prentice Hall of India Pvt. Ltd., New Delhi.

Diana East Cott and Others(1990) : Aspects of Educational and Training Technology. Kogan Page, LONDON, Nichols Publishing Company, New York.

Garret H.E.(1973) : Statistics in Psychology and Education, Vakils, Simon Pvt. Ltd. Sixth U.S. Edition.

Ghosh Pallab(2006): Numerical Methods with Computer Programs in C++, Prentice Hall of India, New Delhi

Guilford, J.P.(1950) : Fundamental Statistics in Psychology and Education, McGraw Hill Book Company, New York.

Jain M.K. & Others (2005) : Numerical Methods (Problems and Solutions), New Age International Publishers, New Delhi.

Langhorne Mary Jo and Others (1989) : Teaching with Computers, Kogan Page, London.

Mukhopadhyay M. (1991): Educational Technology, Vol. I & II, AIAET, New Delhi,

Patel V.A.(1994): Numerical Analysis, Harcourt Brace College Publishing, USA.

Philip Barker and Harry Yeates (1985) : Introducing Computer Assisted Learning, PHI New Jersey.

Rajaram V (1994) : Computer Oriented Numerical Methods, 3^rd Edition, PHI New Delhi.

Rao S. Balachandra & Shantha C.K. (2004): Numerical Methods, University Press (India) Private Limited, Hyderabad (India).

Sharples,M. & Cyclops (1982): A Case Study in the design of User Friendly Educational Technology, Report No. 23, Computer Assisted Learning Research Group, The Open University, Milton Keynes.

About the Authors

Satish Rastogi is M.Sc. (Math), M.Ed., M.A. in Distance Education and Ph.D. in Education. He has 31 years experience in different capacities as an academic. He has published 60 Research papers, 7 Books and produced 13 Ph.D. graduates in Distance Education. Presently he is Professor and Director in Evaluation Division at Yashwantrao Chavan Maharashtra Open University, Nasik – 422 222 (Maharashtra) India.

Ashok S. Pawar is M.Sc. in Electronics Science. He is presently a Selection Grade Lecturer in Physics having 20 years experience at Shri Shivaji Vidya Prasarak Sanstha’s Dr. P.R. Ghogre Science College, Dhule – 424005, (Maharashtra) India.

Computer Assisted Learning (CAL) Strategy for “Numerical Methods Course” - an Experiment